How to Draw in Curvilinear and Cylindrical Perspective Drawing Guide

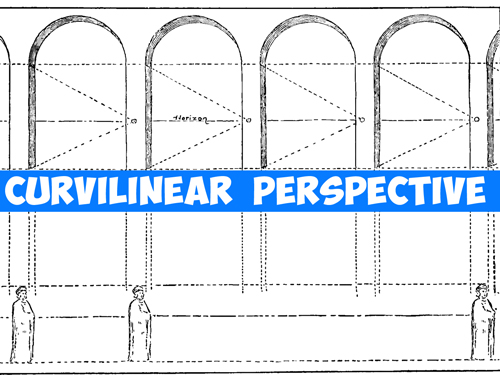
Here is a tutorial on drawing in curvilinear perspective, which is a technique used to create 3-dimensional objects on the surface of your paper. Curvilinear perspective uses either 4, 5, or more vanishing points.  Curvilinear perspective uses curving perspective lines instead of straight converging ones.  This perspective method can use a vertical line as a horizon line … crazy, right?! It can also create both birds’ and worms’ eye views, both at the same time. I found this tutorial in an old drawing book and thought some of you might want to learn about this technique. There isn’t much on curvilinear perspective out there, so I hope that this helps you.

Here are More Perspective Drawing Tutorials

How to Draw in Curvilinear and Cylindrical Perspective Drawing Guide

The actual relations existing between the objects represented are shown in Figure 124…that is, the 3 houses are all in a row. The lines of one have precisely the same directions as those of each of the others, and the fronts of all three are parallel with the fence.

What is shown in figure 125 if we occupied the position which the photographer did when he took the view from that drawing has been made.

Something like it, but with the difference…our eyes would be continually moving, so that the long lines would appear curved, whereas the camera was allowed to remain in one position until one impression was recorded, and then turned in another direction, to remain fixed a little while longer – the result of which is a series of separate and distinct impressions regarding the objects before us.

Now, there can be no doubt about the truth of this last picture, when the conditions under which it was made are understood.

As has just been said, it was drawn from a photograph, and it undoubtedly records just what the observer, who happened also to be a photographer, actually saw.

No greater changes were made in the position of the camera in taking the picture than the observer’s head underwent in looking at the view.

But looking at views is not making pictures, and the question arises as to which is the true picture of the place after all. By which is meant – Which picture produces on the observer’s mind the impression most like that which the place itself would produce?

The impression recorded in Figure 125 from, not one picture, but a series of pictures, made on the inside of a polygonal prism, the surface of which is “developed”, or reduced, to a flat surface afterward. If the impressions were continued without interruption, the record of them would have to be made on the inside of a cylinder which, being unrolled, would give curved where Figure 125 shows jointed straight lines.

The conditions under which the objects represented would in that case be studied, and a comparison of the same with those under which perspective drawings are usually made, are indicated in the diagrams which follow:

Figure 126 shoes the relation existing between a row of arches with square piers, the eye of an observer, and a picture-plane fixed. The dotted lines running from the piers to the station-point show just how wide each pier would have to be in the picture; and, of course, the reader does not need to be told that the piers would all be drawn of the same height, so that the effect would be something like that in Figure 130.

Now, suppose we were to substitute for the picture-plane employed in Figure 126 the curved surface which is shown in Figure 127. The conditions would then be materially changed. But this is precisely the state of things which the revolving camera represents, and which your picture-plane would represent if you kept turning it so as to have it directly in front of you whichever way you were facing.

You will see by the diagram that, as represented in this cylindrical picture, the thickness of the piers as they recede, instead of increasing as it does in Figure 130, remains about the same as that of those nearest to the observer, notwithstanding the face that the more distant piers are seen corner-wise while the nearest ones present hardly more than the width of one face to the observer. And you must also see, that in a picture, every part of which was at the same distance from the observer’s eye, the piers would appear shorter as the piers themselves were farther away. So that, if the observer were to draw on the cylindrical screen shown in Figure 127, and then flatten it out, the result would be like that in Figure 128.

But picture are not usually made on the inside of cylinders, to be unrolled afterward. If they are made on this kind of a surface, as “panoramas” or “cycloramas” are, they are intended to be seen in just that position, and big circular houses are built for their special accommodation – built, too, in such a way that the observer cannot get very far away from the station-point and must see the picture under just the conditions which governed the perspective calculations on which the representation was based.

Figure 128 shows that if we could unroll on of those cylindrical pictures we should find that the representations of all straight lines, except the vertical ones, were really drawn as curves, so that the panorama is said to be drawn in curvilinear perspective; but when seen as they are intended to be seen, they look straight, as they certainly would not do if they had been drawn straight on a flat surface which had been rolled into the form of a cylinder afterward.

Now, on the face of it, the attempt to make flat pictures as if they were cylinders unrolled seems just as
absurd as it would be to try to make cylindrical pictures out of flat ones rolled up. There is more to the
question than this, however, as we shall see.

Any picture- no matter whether it is drawn on a flat surface or on the inside of a cylinder-can appear
quite right only when the observer’s eye-and one eye, at that, remember- is at the station-point.

If you have a cyclorama to exhibit, you can build a little enclosure for the spectator just where he should be, in the middle of the building, and keep him there; and in exhibiting single pictures of considerable importance very much the same thing is often done, but in the vast majority of cases the artist cannot count upon anything of the kind. If he could it would be a very much simpler matter to make pictures than it is.

Remember, however, that if drawn according to the principles of perspective and under conditions which your window screen illustrates the picture will always be correct as long as it is seen from the station point. The eye placed there may wander over the picture as restlessly as it does over the natural scene, and everything represented falls into its place in the one case just as it does in the other. The long lines in Fig 124 foreshorten themselves, and the road and the fence in the foreground taper away to the right and left in the picture just as they do in nature. In fact, the picture cannot help being deceptively right so far as its drawing is concerned as long as the eye is where the artist counted on having it when he made the picture.

Even the question about a good part of the picture being out of focus is one which takes care of itself entirely. One part of the picture is just as much out of focus when the eye is occupied somewhere else as the corresponding part of the natural scene would be. There is not a particle of difference between the two cases.

The artist has to think of something else besides getting his picture in true perspective, because he knows it will be looked at from every other point of view, as well as from the one intended. The chances are, that not one person in a thousand who ever sees it will get himself into the exact position for which its perspective is calculated. A few inches backward or forward, even, will change the whole effect as everybody knows who has tried to draw on the window screen.

Most of us have probably criticized often enough the perspective of the scenery at the theater, not remembering, or not knowing, that it would have been a perfectly easy matter for the painter to make the effect deceptively right for some one eyed man at the back of the house – in the gallery, perhaps at the expense of everybody else in the audience, to each of whom the effect would have been simply ridiculous. He cannot do this and so he introduces a good many modifications which he knows perfectly well to be errors or liberties, for the sake of distributing these inevitable distortions more equitably. In making small pictures the artist’s need not trouble himself much about this; but in large ones it becomes a question of very great moment, and the difficulty has to be solved by drawing all detached objects of any importance as if they formed separate pictures by themselves, which the artist introduces into and reconciles with his composition as a whole the best way he can.

All figures of men and of animals have to be treated in this way; and indeed, nearly all objects not actually connected with other parts of the picture by straight lines may be, and usually are, drawn in this way to the manifest advantage of the whole effect.

Fig 129, which is traced from a photograph of Titian’s Presentation of the Virgin, illustrates this well enough. The buildings are drawn in parallel perspective just as you would draw your neighbor’s house across the way on your window screen, but the drawing of the figures is a different matter altogether.

The following diagram, Figs 130 and 131, shows the kind of effect which would have been obtained if the proportions of the figures had been determined by applying the principles of plane perspective. It simplifies matters to make use of one figure only, determining what its appearance would be in different parts of the picture, rather than to compare it with another figure, and in order that we may determine with considerable precision what its appearance would be under different conditions, this simple architectural feature, an arcade, is introduced to measure its proportions by.

There is just such an arcade at the left hand side of Titian’s picture as you see I have supposed a case in which it should run straight across the whole canvas, the piers being about as thick as these people in the picture, seem to be Then by changing the pillars into persons the monstrosity of figures; drawn according to this principle is demonstrated. The architecture does not look so very bad even in the case of the pier that is farthest to the right but whether it does or not there seems to be little help for it and the master has as you see unhesitatingly drawn

The arcade in Figure 132 is drawn in cylindrical perspective, but the figures into which its piers are transformed (Figure 133) are not much nearer the mark than those in Figure 131. They do not broaden out as they recede, it is true, but they turn their backs on us (or their faces), as it was never intended that they should do; and what is a far more serious matter they grow smaller and smaller as they approach the frame, as they certainly do not in the original picture.

The fact of the matter is, then, that the figures in this picture of Titian’s are not drawn in true perspective either plane or cylindrical, as calculated for a fixed station point. The buildings are, but the figures are not.

These last are drawn, not as they would look when the observer turned his head to look at them, turning his picture at the same time, but as they would look when he moved along so as to be opposite to each in his turn.

This construction, as applied to the arcade is shown in Fig 134, where just as many station points have been used as there were arches to be drawn. It is introduced in this place only for the sake of its application to the human figure; but where simulated architecture indefinitely extended, is employed, as it often is in decoration – on the ceiling of the Sistine Chapel, for example – the same practice has to be resorted to.

Pictures made in this way (and all large figure pieces are so constructed) are records, then, of a succession of impressions obtained from a station point which moves along horizontally before the picture and in a line that is parallel with it, as the visitor to a gallery moves along the rail which keeps him from going too near the pictures, and not from a station point which revolves, as the visitor to the cyclorama has to do.

I have said that large pictures are always made in this way, but it would have been more correct to say that they are usually so constructed.

One is quite at liberty to draw his picture as if it were a cylinder unrolled if he chooses to do so, and that method has occasionally been adopted. It must be admitted, also, that the effect is admirable with certain kinds of subjects – extended landscapes, for instance, in which the principal forms are all at a considerable distance.

A good many of Turner’s landscapes are drawn in this way, and there used to be a small picture of Allston’s in the Boston Museum of Fine Arts to which the same method had been applied.

Both of these masters, moreover, applied the principles of curvilinear perspective. to the straight lines of the architecture as well as to detached objects, which, of course, made the effect very much like that in Figure 125; but this is by no means usual, and in Allston’s case, at least, it is hardly to be regarded in any other light than that of an experiment.

What artists usually do is what Titian has done in the case we have just been regarding,viz. : To draw the architectural part of their subjects in true perspective as applied to a plane like our window screen, and then to add the detached objects, such as figures, as if the observer were standing in front of each in his turn.

All columns and capitals, and a good many other details of buildings, are sufficiently detachable and have enough interest as separate objects to be treated in this way too, and it is customary so to treat them.

Straight lines, like those in pavements walls, lintels, and roofs, have to be left as originally drawn in plane perspective, as they could not otherwise be made to agree with the spectator’s idea of what level things ought to be, as derived from the lines of the room in which the picture was hung and even from its own frame.

And, generally speaking, the connection of all square cornered members with the long lines of the picture is so close that the rule given above has to be observed; thus, a square post will usually be drawn as at A, Fig 136, no matter how awkward the effect may be to an observer who does not occupy the station point. But the circular one which might be made out of it would not, in the same position, be drawn as at B, which a strict regard for perspective would demand, but as at D instead, just as if the square stick from which it might have been made had been drawn as it is either at C or at E. As its lines are curves, there is no difficulty in reconciling them with the straight lines around them. Even the observer at the station point does not notice that the posts are too small and to everybody else they look a great deal better than they would have done if they had been drawn in true perspective.

As has just been said, this treatment has sometimes been extended to the long straight lines of the picture too, very notably by Turner; and the result, where the architectural lines do not come too near the foreground, is certainly very delightful. The charm of it is to be ascribed partly to the picturesque-ness of arrangement into which every detail of the picture necessarily falls when treated in this way, but partly,

also, to the association which a reflective observer discovers between the impression which the picture makes and that which he derives from nature.

Fig 137, Turner’s picture of the “Bridge at Coblentz,” which is taken from Ruskin’s “Elements of Drawing,” is a first rate example of this painter’s application of the principles under discussion.

The eye wanders over such a picture as it does over nature and finds everywhere the same retreating and converging lines, giving an idea of vastness which is not to be obtained in any other way.

Mr. Ruskin has felt the charm of the picture, and has written very beautifully about it, but, curiously enough, he has entirely failed to discover the real motive for the peculiarity of treatment which renders it interesting. He has a great deal to say about the currents of rivers and the desirableness of not building bridges level, but the fact that the whole matter is purely one of perspective effect has somehow eluded him.

A glance at the drawing, however, and a comparison of it with Fig 125, will convince anybody that the curvature of the bridge is to be accounted for on this ground, and on no other.

Mr. Ruskin’s theory, that bridges ought to have the highest arches where the water is deepest, may be a very good one, and Turner may have felt the same way about them for aught we know. But there is nothing in the drawing before us to show that he had any opinion about it one way or the other. At any rate, the drawing of this bridge is not to be accounted for on any such grounds.

The curvature is exaggerated, it is true for an observer would have to stand nearer to the bridge than the rest of the picture indicates, to obtain as much of a curve as this in running his eye along it; but the Fig 136 exaggeration is not a very great matter, after all, and the facts of nature have probably suffered quite as serious misrepresentation in the same picture in many things for which no good Turnerian would ever think of requiring an explanation.

And the fact is not to be denied that in nature the curve is there. You can see it every time you run your eye along the roofs across the street, if you can only disabuse your mind of its preconceived notion that the roofs are level. Indeed, you can hardly see anything else in the lines of the sky, whose dome-like character is purely a matter of perspective effect, the actual curvature that exists in the stratification of all the clouds to be seen from any point on the surface of the earth being perfectly insignificant; and we perceive it, partly because the lines are so very long but partly, also, because we do not have the flatness of the cloud beds impressed upon us every moment of our lives, as we do the straightness of the edges of the buildings As a means of assisting the artist to reproduce the impressions which are made by nature, this modification of details in accordance with the principles of cylindrical perspective is constantly resorted to, unconsciously, it may be, but none the less certainly. Anyone painting an extended view out of doors represents distant objects, not as they would appear if projected upon a fiat screen, but as if they were drawn on the inside of a cylinder, as Turner drew this bridge.

Here are More Perspective Drawing Tutorials

Technorati Tags: curvilinear perspective, 4 point perspective, 5 point perspective, cylindrical perspective